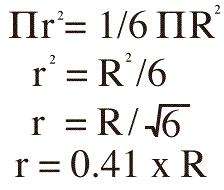|
 Introduction
This unit contains two activities involving splitting regular pentagons into triangles and into smaller pentagons.
These make interesting puzzles.
Activity
I.
[top]
One method of computing the area of a polygon is to partition it into right
triangles, and to compute and add their areas. For example, in this activity,
a regular pentagon can be divided into right triangles or into smaller
pentagons. Such partitions often make very interesting puzzles.
What to do: Divide a regular pentagon into 6 right triangles.
Cut them out, and you have a puzzle that you may put together again.
 Supplies:
Compasses, protractors, rulers, index cards (or triangles) to draw right
angles, colored poster board, stiff construction paper or card stock,
scissors, a simple calculator, and envelopes to keep the puzzles in.
Remarks
1. There are six (!) different solutions to this problem. One was
just discovered by Erica Duran in my Math 313 class spring 2007!
If a student makes more than one puzzle, they must be of different colors;
otherwise the pieces will get hopelessly mixed up.
2. Students should already know how to draw a regular pentagon. Precision in
drawing and cutting is important. The main intellectual challenge is in the
design. If students do not know how to begin, the teacher may show them one
solution.
3. You will see that triangles of the same shape (similar triangles) are used
over and over again, so this lesson may go together with units about area,
similarity, or stars.
Solutions
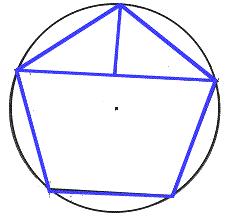
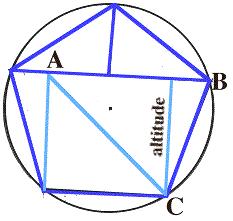
This image shows the first step. A regular pentagon is inscribed in a circle.
The top of the pentagon is divided into two right triangles. This first step
is common to all the solutions presented below. The solutions differ only in
the subdivision of the lower quadrilateral into 4 triangles.
 
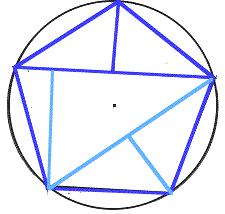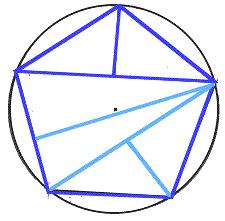
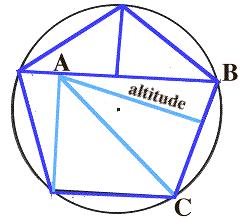
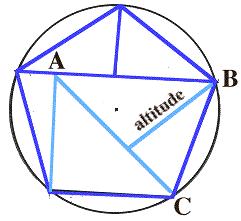
These 3 solutions are obtained by drawing 3 different
heights in the triangle ABC.
  
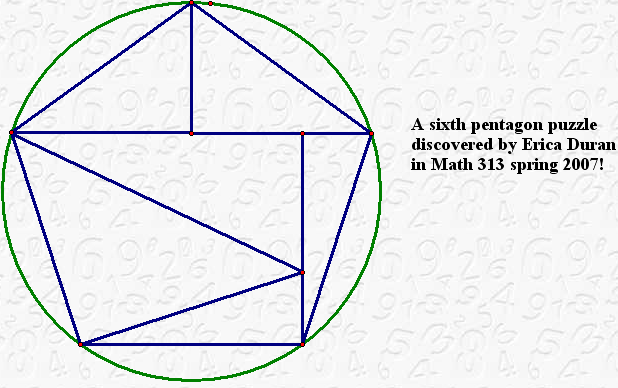
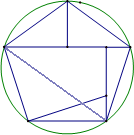
The sixth solution, by Erica Duran, is shown below.
A variant of this unit is to draw the
puzzles and not cut them out.
Activity
II.
[top]
One can also create pentagon puzzles by dividing a regular pentagon into 6
smaller pentagons of equal area.
What to do: Divide a regular pentagon into 6 pentagons. Cut
them out, and you have a puzzle that you may put together again.
Supplies: Compasses, protractors,
rulers, index cards (or triangles) to draw right angles, colored poster
board, stiff construction paper or card stock, scissors, a simple calculator,
and envelopes to keep the puzzles in.
1. Using cardstock, draw a pentagon inside a larger regular pentagon.
The center pentagon should also be a regular pentagon (and thus similar to
the large pentagon).
2. The other five pentagons are not regular. They are all congruent to
each other, and their combined area is 5/6 of the area of the large pentagon.
Do you see a way to do it? If not, see below!
3. After you divide the pentagon, cut it into pieces for a nice
puzzle.
Solutions
We first inscribe a regular pentagon in a circle with radius R. (A radius of
10 cm is very convenient.) Next we construct a concentric circle with radius
r, whose area is 1/6 the area of the larger circle. (See picture below.)
The area
of the large circle (with radius R) is
The area of the small circle (with radius r) is
So
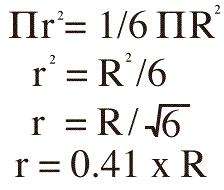
So if the radius R of our large circle is 10 cm, the radius r of our small
circle is 4.1 cm. We inscribe a regular pentagon in the small circle. In our
picture, its edges are not parallel to the edges of the larger pentagon, but
turned 36 degrees (see picture below).
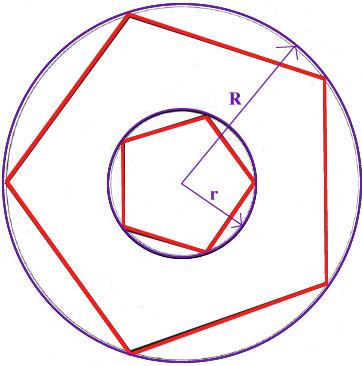
The ratio of the area of an
inscribed pentagon to the area of the circle in which it is inscribed is
constant. So therefore, the ratio of the area of the small pentagon to the
large pentagon is the same as the ratio of the small circle to the large
circle, namely, the fraction 1/6.
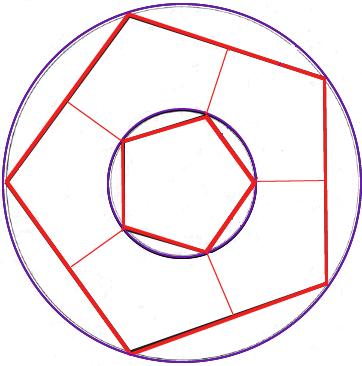
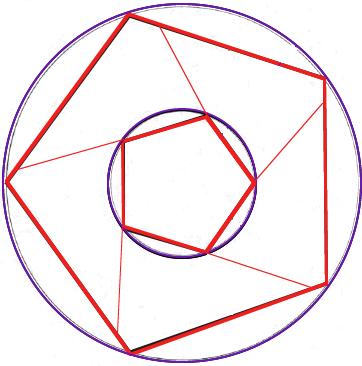
Now the area that is inside the large pentagon and outside the small pentagon
is 5/6 of the area of the large pentagon. We want to divide it into five congruent
pentagons. Did you figure out some ways to do this? There are infinitely
many! Here are some example solutions for you.
 
A sixth pentagon puzzle discovered by Erica Duran in Math 313 spring 2007!
|
