---

Reasoning by analogy: Cutting polyhedra

Our intuitive knowledge of the space around us is poor. We often cannot answer very simple questions about familiar objects without looking at them and handling them. This unit should be taught after students have already made cubes, tetrahedra, and octahedra from poster board (skeletal or solid), and therefore they know their basic properties. These solids should be available to them in this lesson.

First observation.

If we divide a square by cutting it at one half of its height and one half of its width by two straight lines parallel to its sides, we get 4 smaller squares each having an area 1/4 of the original square.

Figure 1

Second observation.

If we divide an equilateral triangle by cutting it at one half of its heights by three straight lines parallel to its sides, we get 4 smaller triangles, each having an area 1/4 of the original triangle.

Figure 2.

Third observation

If we divide a cube by cutting it at one half of its length, width, and height by three planes parallel to its faces, we get 8 smaller cubes, each having a volume of 1/8 of the original cube.

	Original Cube
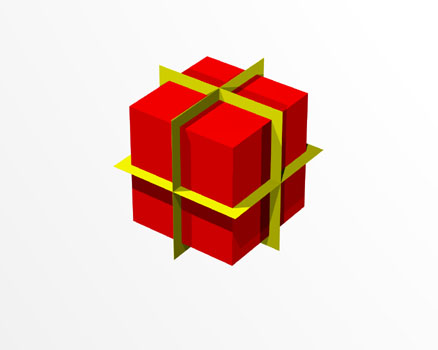	Cube with 3 yellow planes passing through the midpoints of each edge. Each plane is parallel to two of the faces.
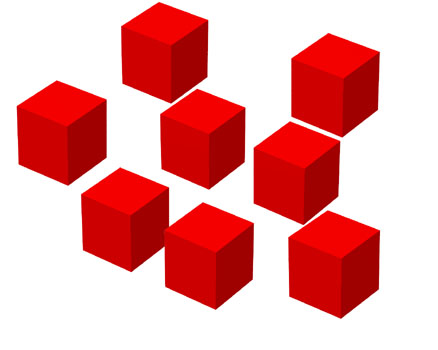	You can see the eight smaller cubes resulting from the decomposition of the main cube

Figure 3

---

Questions.

(A) What are we going to get if we cut a regular tetrahedron at one half of its heights by four planes parallel to its faces?.

(B) What are we going to get if we cut a regular octahedron at one half of its heights by four planes parallel to its faces?

---

Answers.

(A) If you reason by analogy that a tetrahedron splits into smaller tetrahedra, and an octahedron splits into octahedra, you are wrong. The situation is more complex.

A tetrahedron splits into:
4 tetrahedra sitting in the four corners and one octahedron in the middle.
Each tetrahedron has 1/8 of the original volume, and the octahedron has the remaining 1/2.

See why this is true.

(B) An octahedron splits into:
6 octahedra sitting in the six corners and 8 tetrahedra, one in the middle of each face.
Each octahedron has 1/8 of the original volume, and they all add up to 3/4 of the total.
Each tetrahedron has 1/32 of the original volume, and they add up to the remaining 1/4 of the total.

Let's examine this more closely.

An animation to show the different shapes in the octahedron (very large file)

---

Class project

Students make 7 regular octahedra, and 12 regular tetrahedra from poster board, each having edges 1 inch long. The models are then used to demonstrate both of the decompositions discussed above. (Notice that with the same edges, the volume of a tetrahedron is 1/4 the volume of an octahedron.). These are the graphic files with predrawn layouts for the required octahedra and tetrahedra. Both the octahedra and tetrahedra can be put together with tape.